Patrick Graven Geometry
Geometry has always been something I have been interested in from a young age. It is a branch of mathematics concerned with lines, points, shapes, angles, positions and the dimensions of things, it is one of the oldest branches of mathematics. I think of geometry as a mathematical art, with the visuals from its shapes. The word Geometry comes from the combination of the Greek words "Geo" meaning “Earth” and "Metron" meaning “Measure”, for the measurement of the Earth. One of the best known contributors of geometry is Euclid. Euclid was a Greek mathematician best known for his treatise on geometry, known as The Elements. In it, Euclid laid down the rules of geometry. The book of The Elements is one of the most influential mathematical works in history and serves as the main textbook for teaching geometry. The lifelong nature of the book The Elements must make Euclid the leading mathematics teacher of all time, especially when it comes to geometry. However, little is known of Euclid's life except that he taught in Alexandria in Egypt. Euclid was interested in everything you could do with a ruler and a compass. He came up with his own rules, known as Axioms, that described some basic things you could do with these tools, as well as some facts about lines and angles he thought were true and didn't need to be explained. Euclid's book The Elements was the earliest known systematic discussion of geometry and is still one the greatest works of all time. Euclid is often referred to as the 'Father of Geometry'.
Archimedes was a Greek mathematician, one of the best mathematicians that ever lived. His contributions to geometry transformed the subject and the methods that he had anticipated the integral calculus. Archimedes wasn't just a mathematician, he was both a great engineer and a great inventor. He invented a wide variety of machines and made important contributions to the field of mathematics. He perfected the methods of integration and devised formulae to calculate the areas of many shapes and the volumes of many solids. He designed a special group of semi regular polyhedrons, geometric solids whose faces are each flat polygons known as Archimedean solids. These solids, the faces are regular polygons and their sides are all of equal length. Archimedean solids has faces of two or more different types of regular polygons such as pentagons and hexagons. These solids can be positioned inside a sphere so that every vertex makes contact of the surface of the sphere. Although Archimedes achieved recognition by his mechanical inventions, he believed that pure mathematics was the only worthy pursuit. With most of his work, Archimedes used some very practical methods to discover results from geometry, he experimented a lot and applied his theoretical knowledge into practice. The achievements of Archimedes are quite magnificent. Through the medium of geometry, his mathematical proofs, works and inventions revealed such great precision and originality of thought, exhibiting an apex of geometry.
One other great contributor to geometry was the Italian Renaissance polymath, Leonardo da Vinci, whose areas of interest is a vast list of creative activities, including invention, painting, architecture, sculpting, anatomy, science geology, astronomy and geometry. While working on many of his paintings, Leonardo concentrated intensely on geometry. Leonardo was fascinated of the intense relationship between art and visual mathematics. His fascination with the sense of infinity, as depicted in his own drawings and designs, was perhaps an precursor to geometry and their relationship to nature. It is known that one of his friends included the Franciscan friar and mathematician, Luca Pacioli. He was extremely interested in studying polyhedra, which are solids that consist of three dimensions with flat polygonal faces, straight edges and sharp vertices. Pacioli was fascinated by polyhedra, studied their properties, and constructed wooden models for many of the solids. Luca Pacioli collaborated with Leonardo da Vinci on the book De Divina Proportione. In this book, he discussed the concept of “golden proportion” and the properties of different polyhedra. Leonardo studied mathematics and geometry with Pacioli and was more interested in descriptive features of objects. For the polyhedra, Leonardo supplied two views: a plane view and an empty view where he removed the sides to better reveal the complete structure of the polyhedron. To this day, Leonardo's depth of knowledge and his understanding across a wide range of disciplines sets him aside from everyone.
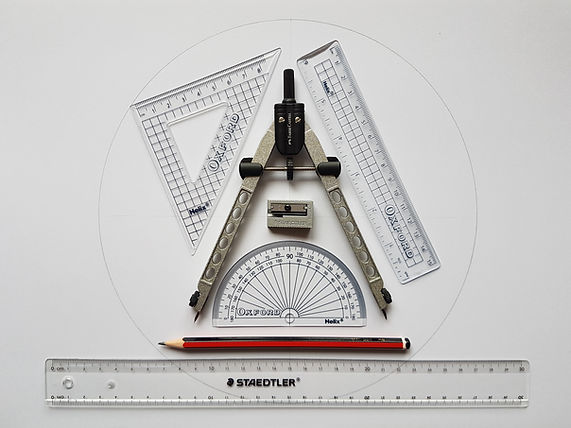
In geometry, polygons are straight sided flat shapes that are close in a space. They are defined by the number of sides that they have. Every polygon has an interior and exterior, is a two dimensional shape and uses only line segments to create its sides. There are two main types of polygons, regular and irregular. To be a regular polygon, every interior and exterior angle in the polygon is equal to every other interior and exterior angle, and every side is equal in length to every other side. To work with polygons, one must know the most common two dimensional curved shape, the circle. In order to work with circles in geometry, it is important to understand the key properties of a circle: a line straight across the centre of a circle is the diameter, half of the diameter is the radius and the line around the edge of a circle is called the circumference. The drawings I do are based on regular polygons, shapes with all equal sides. Some examples of regular polygons are a pentagon, hexagon and an octagon. There are different ways to construct polygons, the way I construct them is from a circle. First I draw a circle with the compass, knowing that in mathematics there are 360° in a whole circle, I divide 360° by the number of sides I want to have, once I know, I draw a vertical line down the centre point of the circle, after I do that, I mark the degrees of the sides that I want with the protractor, when I have that done, I draw a line from the centre point of the circle to all the marking points and then join them all up on the circumference of the circle. This is how I create regular polygons and work from their circumference all the way inward to the centre point of the circle. I join the points of the angles to one another and I work some more with the lines and the intersections that have been created. I join the new intersections and keep working inward until I am content with a design that I want. This is how I create my artwork.